3.8 Derivatives of Logarithmic Functions
Hi, guys. So right now, we're just finding a bunch of ways in which we can make finding derivatives easier. This section, we find out how to find derivatives of logarithmic functions. The lesson itself is pretty short. If you do the derivative of:

you get:

From this, we can generate the general theorem that:

In verbal terms this means that the derivative of the function ln of x equals one divided by x
Don't forget, however, that the chain rule still applies. So if you have this problem for example:

The answer is:

Notice how we first just differentiated the ln of x squared as a whole. Then we differentiated what was inside the parentheses--the x squared itself.
These are very helpful rules because they help us solve many problems that otherwise we wouldn't know how to solve! Like this one!
Find the deriviative of:

How do we do that? With the natural log derivative theorem of course! There are several steps to applying this rule to a problem. These steps are also a general rule of thumb that you can use with any logarithmic equation.
1) Take the natural log of both sides
So:

All we did was take the natural log of both sides
2) Simplify with the help of logarithm laws.
Don't forget from last year that there are several other rules about derivatives that we can use to help us. First off, remember that:

Oh look! We just happen to have the log of a quotient! Perfect!
So this means that our new result is:

The other logarithm rule we should remember is:

This helps us to since we have a a logarithm of a product. So now we have:

Now don't forget the rule where you can take the exponent of a logarithmic function and put it in front of the logarithm. Like this:

So since each of our three terms have exponents, we can just put those exponents in front of each term like so:

3) Use implicit differentiation for x
We know that:

So now we can apply this to our problem. Remember, don't forget to use the chain rule to those that have more than just x inside the ln ( ).

See where we had to use the chain rule? The 2x is the derivative of the x squared plus one and the 3 is the derivative of 3x + 2.
Notice also that we had to differentiate the y also. We had to use chain rule for that too, since 1 over y is the first term, and then we're still left with the y, which we had to differentiate by itself, giving us y prime.
4) Solve for y prime

Oh, but what does y equal? We know from the original equation. So we can go ahead and substitute that in, giving us our final equation:

Yayyy! We did it!
Here's something cool, guys. It's a very simply step-by-step proof of how they "derived" the formula for finding derivatives of logarithmic functions. Enjoy!
http://ltcconline.net/greenl/courses/116/ExpLog/logDerivative.htm
I thought these comics were funny. Mr. French, I couldn't remember if you had the amazing plusman on your wall or not. So I put it up anyway.



You're next, Laurie. And then the cycle starts all over!







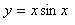


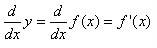













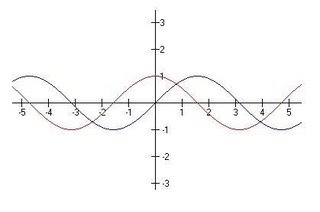
 Now, let's do the same thing to the f(x)=cos(x) graph in order to find the its derivative.
Now, let's do the same thing to the f(x)=cos(x) graph in order to find the its derivative. After making the derivative graph (in red), you get this:
After making the derivative graph (in red), you get this: Incredible. It looks to me that the derivative of the cosine graph is:
Incredible. It looks to me that the derivative of the cosine graph is:




