Section 2.3: Calculating Limits Using the Limit Laws

Hey guys! So bear with me, I’m not sure how great this will be…



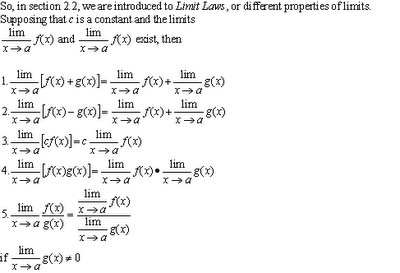
Verbally, it goes something like this:

1. The limit of a sum is the sum of the limits (Sum Law)
2. The limit of a difference is the difference of the limits (Difference Law)
3. The limit of a constant ( c ) times a function is the constant times the limit of the function (Constant Multiple Law)
4. The limit of a product is the product of the limits (Product Law)
5. The limit of a quotient is the quotient of the limits (provided that the limit of the denominator is not equal to zero) (Quotient Law)

(Ok, so that all makes sense, right? Don’t worry, because in the next section we will prove Law Numero Uno. The other proofs are in an appendix somewhere. For fun with proofs on this stuff, as well as more info on this leson, go to chapter 2 on this page)



But wait, there’s more!







So far, all of the limits make sense, right? It’s all pretty intuitive.



Oh man. Ok, the book tells me that that’s it for the laws. However, we are not yet done. We have miles to go before we sleep (ok, maybe not miles, just hours)








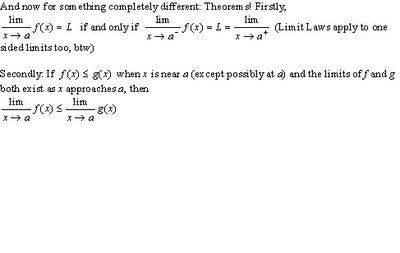
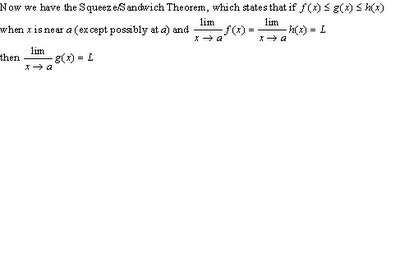
Ok, there you go! Now, before my brain shuts off entirely from exhaustion, allow me to present a sample problem.



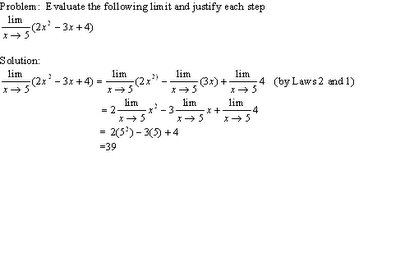
Ta-da!

Oh, and by the by, Taylor, you're on deck...

Sorry about the huge gaps, you guys...I didn't crop it properly when I re-formatted the equations! My apologies!